Cho tam giác ABC. Xác định I sao cho 3 vecto IA -- 2 vecto IB + vecto IC
Câu hỏi:
Cho tam giác ABC. Xác định I sao cho \[3\overrightarrow {IA} - 2\overrightarrow {IB} + \overrightarrow {IC} = \overrightarrow 0 \] (\[\overrightarrow {IA} ,\overrightarrow {IB} ,\overrightarrow {IC} ,\overrightarrow 0 \] là các vectơ).
Trả lời:
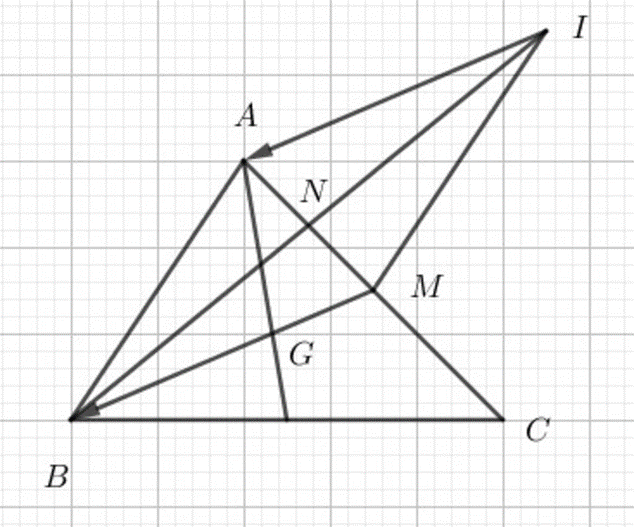
Gọi M là trung điểm cạnh AC
G là trọng tâm ΔABC ⇒ \(\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} = \overrightarrow 0 \) và \[\overrightarrow {BG} = \frac{2}{3}\overrightarrow {BM} \]
Ta có: \[3\overrightarrow {IA} - 2\overrightarrow {IB} + \overrightarrow {IC} \]
\[ = \overrightarrow {IA} + \overrightarrow {IB} + \overrightarrow {IC} + 2\overrightarrow {IA} - 3\overrightarrow {IB} \]
\[ = \overrightarrow {IG} + \overrightarrow {GA} + \overrightarrow {IG} + \overrightarrow {GB} + \overrightarrow {IG} + \overrightarrow {GC} + 2\overrightarrow {IA} - 3\overrightarrow {IB} \]
\[ = 3\overrightarrow {IG} + 2\overrightarrow {IA} - 3\overrightarrow {IB} \]
\[ = - 3\left( {\overrightarrow {GI} + \overrightarrow {IB} } \right) + 2\overrightarrow {IA} \]
\[ = - 3\overrightarrow {GB} + 2\overrightarrow {IA} = \overrightarrow 0 \]
⇒ \[\overrightarrow {IA} = \frac{3}{2}\overrightarrow {GB} = \frac{{ - 3}}{2}\overrightarrow {BG} = \frac{{ - 3}}{2}.\frac{2}{3}.\overrightarrow {BM} = - \overrightarrow {BM} = \overrightarrow {MB} \]
⇒ \[\overrightarrow {IA} = \overrightarrow {MB} \]
Tứ giác ABMI là hình bình hành
Gọi N là trung điểm cạnh AM
Lấy I đối xứng với B qua N ta được điểm I thỏa mãn đề bài.
